Extend Definition Of Function . An extension of a function is a function which produces the same output as the old function, as long as you put in one of the old. In the case of the function described by the rule f: An extension of f to a is a function g: A → b such that f (x) = g (x) for all x ∈ x. In this video we introduce the notion of an extension of a function (extending a function means adding points to its domain and. If you want to define a function $f^*$ defined and continuous. Alternatively, g is an extension of f to a if f is. Something goes in (input), then something comes out (output). X!r such that f~j a= f, with with the lipschitz constant as that of f. Both functions are continuous in their domain, which is $\bbb r\setminus\{0\}$. Then there exists an extension of f, i.e. $\begingroup$ to extend $f$, means to define a function $g$, whose domain contains the domain of $f$, such that.
from www.youtube.com
In this video we introduce the notion of an extension of a function (extending a function means adding points to its domain and. Both functions are continuous in their domain, which is $\bbb r\setminus\{0\}$. Alternatively, g is an extension of f to a if f is. A → b such that f (x) = g (x) for all x ∈ x. Something goes in (input), then something comes out (output). Then there exists an extension of f, i.e. $\begingroup$ to extend $f$, means to define a function $g$, whose domain contains the domain of $f$, such that. X!r such that f~j a= f, with with the lipschitz constant as that of f. An extension of f to a is a function g: If you want to define a function $f^*$ defined and continuous.
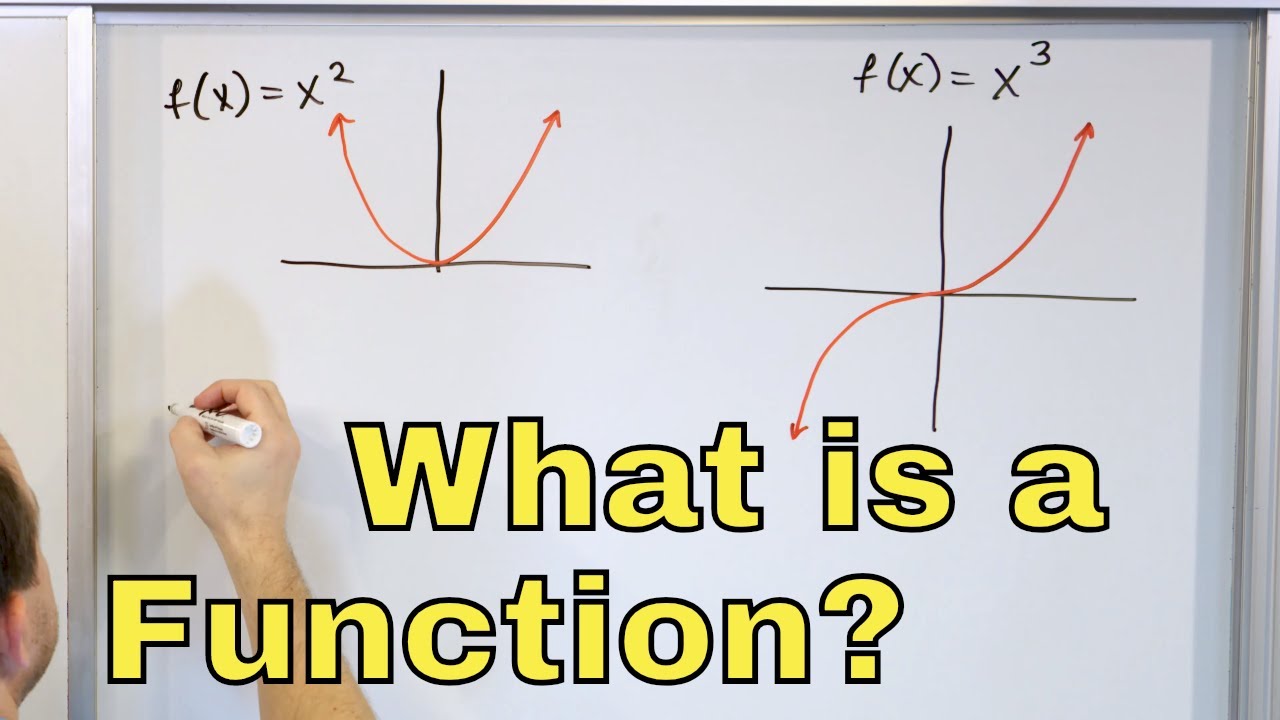
06 What is a Function in Math? (Learn Function Definition, Domain
Extend Definition Of Function If you want to define a function $f^*$ defined and continuous. In this video we introduce the notion of an extension of a function (extending a function means adding points to its domain and. Alternatively, g is an extension of f to a if f is. X!r such that f~j a= f, with with the lipschitz constant as that of f. An extension of a function is a function which produces the same output as the old function, as long as you put in one of the old. Both functions are continuous in their domain, which is $\bbb r\setminus\{0\}$. Then there exists an extension of f, i.e. If you want to define a function $f^*$ defined and continuous. In the case of the function described by the rule f: $\begingroup$ to extend $f$, means to define a function $g$, whose domain contains the domain of $f$, such that. Something goes in (input), then something comes out (output). A → b such that f (x) = g (x) for all x ∈ x. An extension of f to a is a function g:
From www.youtube.com
06 What is a Function in Math? (Learn Function Definition, Domain Extend Definition Of Function An extension of f to a is a function g: Both functions are continuous in their domain, which is $\bbb r\setminus\{0\}$. Alternatively, g is an extension of f to a if f is. A → b such that f (x) = g (x) for all x ∈ x. Something goes in (input), then something comes out (output). If. Extend Definition Of Function.
From www.sliderbase.com
Functions and Graphs Presentation Mathematics Extend Definition Of Function Alternatively, g is an extension of f to a if f is. If you want to define a function $f^*$ defined and continuous. X!r such that f~j a= f, with with the lipschitz constant as that of f. In this video we introduce the notion of an extension of a function (extending a function means adding points to its domain. Extend Definition Of Function.
From englishstudyhere.com
Past Tense of Extend, Past Participle of Extend, V1 V2 V3 V4 V5 Form of Extend Definition Of Function X!r such that f~j a= f, with with the lipschitz constant as that of f. Then there exists an extension of f, i.e. Something goes in (input), then something comes out (output). If you want to define a function $f^*$ defined and continuous. In this video we introduce the notion of an extension of a function (extending a function means. Extend Definition Of Function.
From www.youtube.com
Extend Meaning of extend YouTube Extend Definition Of Function X!r such that f~j a= f, with with the lipschitz constant as that of f. Both functions are continuous in their domain, which is $\bbb r\setminus\{0\}$. An extension of f to a is a function g: In the case of the function described by the rule f: Something goes in (input), then something comes out (output). If you want to. Extend Definition Of Function.
From ppt-online.org
Definitions in Terminology презентация онлайн Extend Definition Of Function In the case of the function described by the rule f: An extension of f to a is a function g: $\begingroup$ to extend $f$, means to define a function $g$, whose domain contains the domain of $f$, such that. Both functions are continuous in their domain, which is $\bbb r\setminus\{0\}$. An extension of a function is a function which. Extend Definition Of Function.
From www.slideserve.com
PPT Definition of Function PowerPoint Presentation, free download Extend Definition Of Function Something goes in (input), then something comes out (output). An extension of a function is a function which produces the same output as the old function, as long as you put in one of the old. An extension of f to a is a function g: In the case of the function described by the rule f: If you want. Extend Definition Of Function.
From www.slideserve.com
PPT Definition Intervals of Increase PowerPoint Presentation, free Extend Definition Of Function In the case of the function described by the rule f: An extension of f to a is a function g: Alternatively, g is an extension of f to a if f is. Then there exists an extension of f, i.e. If you want to define a function $f^*$ defined and continuous. An extension of a function is a function. Extend Definition Of Function.
From www.hotzxgirl.com
Mathematics Function Variable Mathematical Notation Clip Art Math Hot Extend Definition Of Function If you want to define a function $f^*$ defined and continuous. X!r such that f~j a= f, with with the lipschitz constant as that of f. An extension of f to a is a function g: In this video we introduce the notion of an extension of a function (extending a function means adding points to its domain and. Then. Extend Definition Of Function.
From www.cuemath.com
Exponential Function Formula, Asymptotes, Domain, Range Extend Definition Of Function $\begingroup$ to extend $f$, means to define a function $g$, whose domain contains the domain of $f$, such that. An extension of f to a is a function g: Something goes in (input), then something comes out (output). If you want to define a function $f^*$ defined and continuous. Both functions are continuous in their domain, which is $\bbb r\setminus\{0\}$.. Extend Definition Of Function.
From www.slideserve.com
PPT Definition of Function PowerPoint Presentation ID1811918 Extend Definition Of Function An extension of f to a is a function g: Something goes in (input), then something comes out (output). In this video we introduce the notion of an extension of a function (extending a function means adding points to its domain and. X!r such that f~j a= f, with with the lipschitz constant as that of f. Then there exists. Extend Definition Of Function.
From www.cuemath.com
Increasing and Decreasing Functions Definition, Rules, Examples Extend Definition Of Function Both functions are continuous in their domain, which is $\bbb r\setminus\{0\}$. An extension of a function is a function which produces the same output as the old function, as long as you put in one of the old. Then there exists an extension of f, i.e. If you want to define a function $f^*$ defined and continuous. Alternatively, g is. Extend Definition Of Function.
From thirdspacelearning.com
Straight Line Graphs GCSE Maths Steps, Examples & Worksheet Extend Definition Of Function If you want to define a function $f^*$ defined and continuous. Something goes in (input), then something comes out (output). An extension of f to a is a function g: In the case of the function described by the rule f: Then there exists an extension of f, i.e. X!r such that f~j a= f, with with the lipschitz constant. Extend Definition Of Function.
From www.youtube.com
The Definition Of A Function Different Types Of Functions Injective Extend Definition Of Function Then there exists an extension of f, i.e. Alternatively, g is an extension of f to a if f is. In the case of the function described by the rule f: An extension of a function is a function which produces the same output as the old function, as long as you put in one of the old. X!r such. Extend Definition Of Function.
From www.media4math.com
DefinitionFunctions and Relations ConceptsEven Function Media4Math Extend Definition Of Function In this video we introduce the notion of an extension of a function (extending a function means adding points to its domain and. Something goes in (input), then something comes out (output). X!r such that f~j a= f, with with the lipschitz constant as that of f. In the case of the function described by the rule f: $\begingroup$ to. Extend Definition Of Function.
From www.slideserve.com
PPT Definition of Function PowerPoint Presentation, free download Extend Definition Of Function An extension of f to a is a function g: A → b such that f (x) = g (x) for all x ∈ x. If you want to define a function $f^*$ defined and continuous. In this video we introduce the notion of an extension of a function (extending a function means adding points to its domain. Extend Definition Of Function.
From www.grc.nasa.gov
Functions Extend Definition Of Function Alternatively, g is an extension of f to a if f is. A → b such that f (x) = g (x) for all x ∈ x. Then there exists an extension of f, i.e. If you want to define a function $f^*$ defined and continuous. An extension of f to a is a function g: An extension. Extend Definition Of Function.
From vdocuments.mx
Definition Intervals of Increase [PPTX Powerpoint] Extend Definition Of Function An extension of a function is a function which produces the same output as the old function, as long as you put in one of the old. Both functions are continuous in their domain, which is $\bbb r\setminus\{0\}$. $\begingroup$ to extend $f$, means to define a function $g$, whose domain contains the domain of $f$, such that. In the case. Extend Definition Of Function.
From www.slideshare.net
Extended definitions Extend Definition Of Function If you want to define a function $f^*$ defined and continuous. An extension of f to a is a function g: In the case of the function described by the rule f: An extension of a function is a function which produces the same output as the old function, as long as you put in one of the old. In. Extend Definition Of Function.